Month: March 2016

Galileo’s Amazing Trek to Jupiter

joecamatoLeave a comment

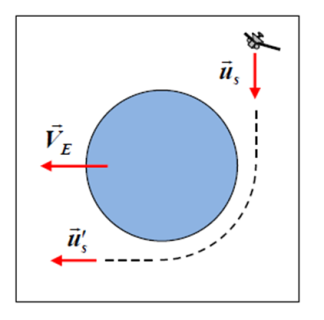
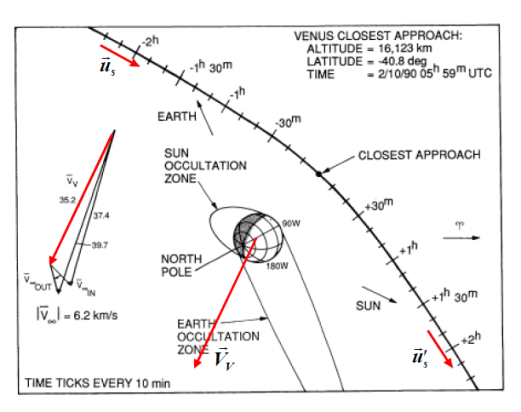
Jonathan Levine, who is presently teaching the introductory mechanics course at Colgate (Spring 2016), has pointed out that the gravity-assist maneuver described in Problem 5.21 is hopelessly unrealistic.  If executed as described, the spacecraft Galileo would leave the vicinity of Venus with a heliocentric speed of 107 km/s, more than twice the speed needed to escape the solar system!  Galileo’s actual fly-bys of Venus and Earth were described in a paper by D’Amario, Bright and Wolf, “Galileo Trajectory Design,” Space Science Reviews 60, 23-78 (1992).  The figures below are adapted from that reference.  The first shows the fly-by of Venus, which took place on February 10, 1990.

Galileo Fig. 1

 

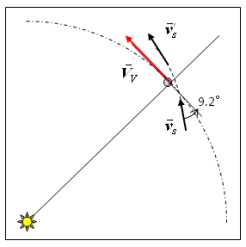Galileo Fig. 2According to the numbers given in the figure above, the spacecraft approached Venus (Vv = 35.2 km/s) with a relative speed \large u_{s}=6.2 km/s.  By using the dot product, we find that the angle between \large \vec{u}_{s} and \large \vec{V}_{V} was 74°, and the angle between \large \vec{{u}'}_{s} and \large \vec{V}_{V} was 47°.  Or, using the law of sines, we can calculate the angle θ between \large \vec{V}_{V} and \large \vec{v}_{s} (the heliocentric approach velocity), and the angle θ’ between \large \vec{V}_{V} and \large \vec{{v}'}_{s} (the spacecraft’s velocity after the fly-by): θ = 9.2° and θ’ = 6.6°.   The velocity vector diagram shows clearly that to increase the speed of the spacecraft, its trajectory must be turned toward the direction of the planet’s velocity.  Here are some additional homework/exam exercises suitable for Chapter 5.

  1. Galileo approached Venus with a heliocentric speed \large v_{s}=37.4 km/s at an angle \large \theta =9.2^{\circ} relative to the direction of \large \vec{V}_{V}=-35.2\hat{i} km/s.  Find the relative velocity \large \vec{u}_{s} (in \large \hat{i},\hat{j} coordinates).  (Ans: \large \vec{u}_{s}=-1.7\hat{i}+6.0\hat{j} km/s)
  2. The encounter with Venus turned Galileo’s velocity closer to the direction of \large \vec{V}_{V}. The angle θ’ between the final velocity\large \vec{{v}'}_{s} and \large \vec{V}_{V} was just 6.6°.  Find the spacecraft’s heliocentric speed just after the fly-by.  (Ans: 39.7 km/s)
  3. Calculate the angle φ between \large \vec{u}_{s} and \large \vec{V}_{V}, and the angle φ’ between \large \vec{{u}'}_{s} and \large \vec{V}_{V}.  Sketch the fly-by in the CM reference frame.  (Ans: \large \varphi =74^{\circ}, \large {\varphi}' =47^{\circ})

The next exercise is suitable for Chapter 10.

4. At its closest approach, Galileo was 16,100 km above the surface of Venus. What was the maximum speed of the spacecraft in the CM reference frame during the fly-by?  (Ans:\large u_{s}(max)= 8.2 km/s)

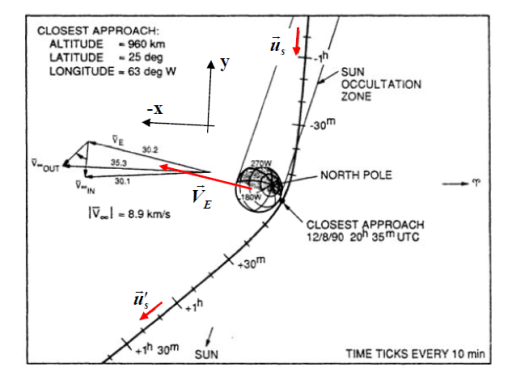
Galileo’s first Earth fly-by took place on December 8, 1990, and is depicted in the figure below (again adapted from D’Amario et al).  We can ask the same set of questions using the numbers given in the velocity vector diagram (insert).  For your convenience, we’ve calculated the relevant angles: \large \theta ,{\theta }'=99.1^{\circ},61.7^{\circ} and \large \varphi ,{\varphi }'=17.0^{\circ},12.8^{\circ}, and the maximum speed relative to Earth is 13.7 km/s.

Galileo Fig. 3

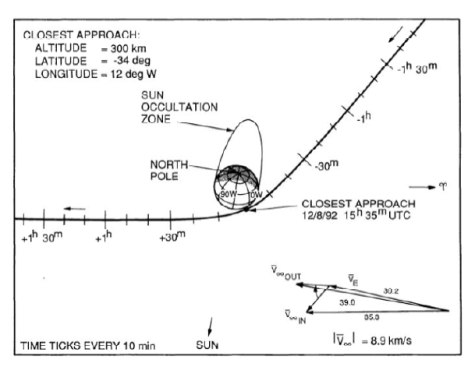
Galileo’s second Earth fly-by took place on December 8, 1992.  Its trajectory in the CM reference frame is shown below.  We’ll leave it to you to calculate the angles from the data given in the velocity vector diagram below.

Galileo Fig. 4

Alert students may wonder why all this fuss was necessary to increase the spacecraft’s speed by a few kilometers per second.  Questions 5 and 7 address this issue.

5. The on-board fuel carried by Galileo was monomethylhydrazine (MMH) oxidized with nitrogen tetroxide (N2O4). The exhaust speed is 3.35 km/s.  If the spacecraft’s mass  without fuel is 2400 kg, find the fuel mass needed to increase its speed by 2.3 km/s (the ∆v achieved by the Venus fly-by).  (Ans: 2400 kg!)

Students can tackle question 6 after studying Chapter 10.

6. Suppose NASA wanted to send Galileo directly to Jupiter, with no gravity-assist  maneuvers, using a Hohmann transfer orbit. What is the speed needed to inject the spacecraft into this interplanetary orbit from Earth?  Neglect the gravitational influence of either planet.  (Ans: 38.6 km/s.  Note that this is the final speed of the spacecraft after its second fly-by of Earth, as shown in the last figure above.)

7. Calculate the fuel mass needed to increase Galileo’s speed from 30 km/s (Earth’s orbital speed) to 39 km/s, the speed needed to reach Jupiter directly from Earth orbit, without gravity-assists.  Let the mass of the spacecraft, depleted of fuel, be 2400 kg, and assume  the exhaust fuel velocity is 3.35 km/s.  (Ans:\large m_{fuel}\approx 14.7M_{spacecraft}\approx 35,000 kg!)

The next question is suitable after studying Chapter 12.  The numbers have been modified to make computation easier.

8. Galileo made two consecutive fly-bys of Earth on its way to Jupiter. On its first fly-by, it     approached Earth with a relative speed \large u_{s}=8.9 km/s at (roughly) a 90° angle from the planet’s velocity.  If the spacecraft’s velocity (in the CM frame) had been deflected by 90°, making its final velocity \large \vec{{u}'}_{s} parallel to \large \vec{V_{E}}, its final speed would have been sufficient to reach Jupiter. (Check this.)  Why was the second fly-by needed?  (Hint: what is the maximum possible deflection?  See section 12.9.   (Ans: To achieve a 90° deflection, the impact parameter  \large s=0.8R_{E}, i.e., the spacecraft would have crashed into Earth.  The maximum possible deflection is 53°.)

Galileo Fig. 5